Vector Field Socks

Based on this video:

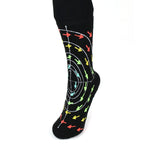
A classic example for student of differential equations is the non-linear pendulum. These socks illustrate what's known as the "phase space" of that system (see the video above), but represented on a cylinder, which is much more natural than most other diagrams you'll find.

One axis represents the angle of the pendulum, and the other represents angular velocity.  But that angle is cyclic; rotating the pendulum 360 degrees doesn't change how it looks.  So the most honest phase space of this pendulum should have this x-axis wrapping round onto itself, as it does on these socks.

The white spiral in this diagram shows a typical trajectory for a pendulum, slowly rotating back and forth as it looses energy and tends towards a state where the angle and angular velocity are both zero.